题 目1：Theoretical study of exceptional points in non-Hermitian free-fermion systems
题 目2：Theory of arbitrary order effective perturbation spin model of arbitrary Hubbard Model
报告人：邹粤禺 中山大学
邀请人：徐刚教授
时 间：2024年1月15日（周一）16:30
地 点：强磁场中心C204会议室
报告摘要1：
Non-Hermitian quantum systems cannot possess both real energy spectrum and orthodontically complete eigenvector set. In previous studies, people focused on the energy spectrum of non-Hermitian quantum systems. For example, we can judge PT symmetry and PT symmetry breaking by the energy spectrum of PT symmetric systems. The topological performance of non-Hermitic systems is judged by the occurrence of zero energy points in the real energy spectrum. However， non-unitary of non-Hermitian system is merely studied. From the insight of Lee-Wolfenstein inequality, we define a generalized Petermann factor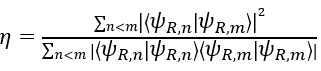 
in our work. Through our computations and research across various non-Hermitian quantum systems, we have discovered a close association between the generalized Petermann factor 
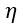and exceptional points. Specifically, discontinuities in 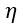correspond to boundary state exceptional points, while discontinuities in the first derivative of 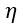correspond to bulk state exceptional points.
报告摘要2：
The theoretical models of magnetic materials are usually spin models such as Heisenberg model. The more essential origin of magnetism is the Coulomb interaction between electrons, which is usually described by Hubbard model in theoretical models. Compared with Heisenberg model, Hubbard model has more essential connection with materials and interaction models. In our work, through the study of the perturbation effective theory, we give the theoretical and practical scheme for deriving the effective spin form of arbitrary expansion order of arbitrary Hubbard model. Besides, In the study of the perturbation effective spin form of Hubbard model, we derive the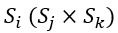 
term in the triangle lattice with magnetic flux, which is of interest in the spin liquid topic. The application of a magnetic field in the diagonal direction of the lattice can lead to the realization of a minimal Toric Code model, which has been experimentally verified by Jian-Wei Pan’s research group.
报告人简介：
Researcher Yue-Yu Zou graduated as a undergraduate in Huazhong University of Science and Technology in 2020 and finished his graduated research in Sun Yat-sen University. During his undergraduate studies, he focused on quantum Hall effect and theory of topological states of matter. His graduate research topics include: 1. Investigating the relationship between non-unitarity and exceptional points of non-Hermitian physics; 2. Attempting to find experimental plans or materials that could realize topological orders such as Toric Code model through the study of arbitrary single-particle Hamiltonian + Hubbard term models.
